First of all, we set up a large metal disk on a central shaft, and made a appropriate measurements of the rotating part of the apparatus and determined its moment of inertia.
And then, for judging the result is correct or not, we connected this apparatus to a 500 g dynamics cart. The cart would drag the string that connected around the disk with constant force in order to give a constant angular acceleration to the disk. Later, we let the cart roll down an inclined track for a distance of one meter, and we could determine the time it took so that we could use those data to figure out the physical moment of inertia.
In the first section, we need to know the mass and radius of the disk to get the inertia, and we measured the radius of the disk in two parts. The bigger part's radius is 0.03 cm, and the smaller part's radius is 1.67 cm. For the mass, we determined the bigger one is 4.08 kg, and the smaller one is 0.749 kg. After this, from below equation, we got the smaller inertia is 0.0001044 kg*m^2, and the bigger one is 0.020115 kg*m^2, so the total inertia is 0.0202 kg*m^2.
And then, we need to know the friction torque of the disk, so we let the disk rotate and stop by itself for measuring the slowing angular acceleration by computer and camera.
Let radius times the acceleration to get the angular acceleration is -2.1208 rad/s^2, for friction torque, we let the angular acceleration times inertia, and we got -0.042841 N*m.
After we measure the angle of the track and the ground, we substitute all data to above equation, so we got the cart acceleration is 0.152 m/s^2.
For one meter inclined track, we got the time is 2.565 s.
However, our disk was not perfect to rotating and it made noise sound, so the time it took was longer the 2.565 s. But we found a good way to make it better is making the angle between ground and track to be bigger, and the error would be smaller even though it was still not perfect.
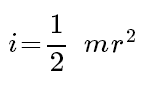
没有评论:
发表评论